AS1 - DNS/LES of the infinite cylinder at Re=3900
Test case leader:
ZJ Wang, University of Kansas
Contributors:
Andrea Beck (University of Stuttgart); Peter Vincent and Brian Vermeire (Imperial College)
Contact:
zjw ku [dot] edu (zjw[at]ku[dot]edu)
ku [dot] edu (zjw[at]ku[dot]edu)
info cenaero [dot] be (info[at]cenaero[dot]be)
cenaero [dot] be (info[at]cenaero[dot]be)
Summary:
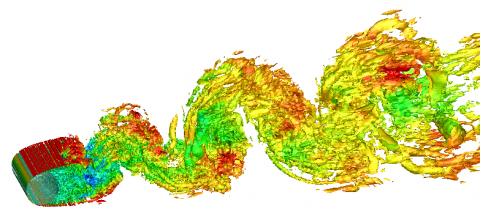
This test case concerns the flow over an infinitely long cylinder at a Reynolds number of 3900, which is simulated using a spanwise periodic mesh. This test case is aimed at characterizing the accuracy and efficiency of high order solvers for the prediction of complex unsteady multi-scale problems under low Reynolds number conditions.
An infinitely smooth initial condition with a bias in the spanwise and vertical direction is imposed. Two flow regimes are of interest for the results:
- Deterministic phase. The unsteady solution at a predefined time t1, before any chaos or turbulence starts, will be used to assess the spatial and time accuracy of the flow solver. The bias is designed to ensure that the non-symmetric flow is not a consequence of round-off or truncation errors. Since this period is expected to be relatively small, hp-refinements or adaptations can be employed to obtain accurate Cl and Cd (error < 0.01 count) values to assess solution spatial and temporal accuracy;
- Turbulent flow. After the flow reaches a statistically periodic state at t2, averaged quantities and Reynolds stresses are computed until a given time t3 for comparison purposes.
Three meshes with different resolutions are provided; participants may use hp-adaptation in the first phase.
Features and challenges:
Curved geometry
Unsteady flow
Subsonic flow
Transitional flow
Direct Numerical Simulation (DNS)
Large Eddy Simulation (LES)