C1 - DLR F11

image1

Test case leader: 

Tobias Leicht, seconded by R. Hartmann, DLR

Contact: 

tobias [dot] leichtatdlr [dot] de (tobias[dot]leicht[at]dlr[dot]de)

info [dot] hiocfd4atcenaero [dot] be (info[dot]hiocfd4[at]cenaero[dot]be)

ralf [dot] hartmannatdlr [dot] de (ralf[dot]hartmann[at]dlr[dot]de)

Summary: 

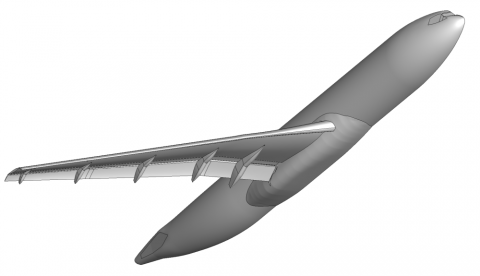
The DLR F-11 high lift configuration was part of the 2nd phase of the AIAA High Lift Prediction Workshop and was extensively investigated with 2nd-order state-of-the-art codes (http://hiliftpw.larc.nasa.gov/index-workshop2.html). The geometry considered for this meshing challenge is designated as configuration 4 in the original workshop and contains slat tracks and flap track fairings (see image).

The geometry is representative for a wide-body commercial aircraft with a classical three element high lift system at the wing leading and trailing edge in a landing setting. The experimental data used for the validation in the framework of HiLiftPW-2 have been measured in the atmospheric low speed wind tunnel of Airbus-Deutschland. Results of grid convergence studies using 2nd order industrial methods are available for structured and unstructured meshes for Mach number M = 0.175, Reynolds number conditions Re = 15.1 x 106 and angles of attack AoA=7°, 16° and 18.5°.

Two types of contributions are expected:

  • Meshes:  participants are expected to demonstrate a methodology to generate hybrid curved meshes, including high aspect ratio extrusion boundary layers, with at least a quadratic representation of the boundary. A series of meshes following specifications by the test case organiser should be provided by March 6th.
  • Computations: participants are expected to provide a single simulation for each of the three conditions. The required data follows the specification of the aforementioned workshop.

Quadratic curved hybrid mesh (3.5e6 elements: prism, pyramids and tetrahedra) by Harlan McMorris from CentaurSoft, can be provided in Gmsh or CGNS format upon request (ralf [dot] hartmannatdlr [dot] de (ralf[dot]hartmann[at]dlr[dot]de)).

Features and challenges: 

Curved geometry

Mesh generation

Subsonic flow

Turbulent flow

Reynolds Averaged Navier Stokes (RANS)

Files
Meshes, geometry and data (stp) (11.09 MB)